1 PHYSICAL QUANTITIES AND UNITS

1.1 PHYSICAL QUANTITIES AND QUANTITY CALCULUS

The value of a physical quantity Q can be expressed as the product of a numerical value {Q} and a unit [Q] $Q = \{Q\}[Q] \quad (1)$ Neither the name of the physical quantity, nor the symbol used to denote it, implies a particular choice of unit. Physical quantities, numerical values, and units may all be manipulated by the ordinary rules of algebra. Thus we may write, for example, for the wavelength of one of the yellow sodium lines $\lambda = 5.896\ \times 10^{−7}\ \rm{m} = 589.6\ \rm{nm} \quad (2)$ where m is the symbol for the unit of length called the metre (see Section 2.2, p. 11), nm is the symbol for the nanometre, and the units metre and nanometre are related by $1\ \rm{nm} = 10^{−9}\ \rm{m}\ \rm{or}\ \rm{nm} = 10^{−9}\ \rm{m} \quad (3)$ The equivalence of the two expressions for $\lambda$ in Equation (2) follows at once when we treat the units by the rules of algebra and recognize the identity of 1 nm and $10^{−9}\ \rm{m}$ in Equation (3). The wavelength may equally well be expressed in the form $\lambda/\rm{m} = 5.896\ \times 10^{−7}\ \rm{or}\ \lambda/\rm{nm} = 589.6 \quad (4)$ It can be useful to work with variables that are defined by dividing the quantity by a particular unit. For instance, in tabulating the numerical values of physical quantities or labeling the axes of graphs, it is particularly convenient to use the quotient of a physical quantity and a unit in such a form that the values to be tabulated are numerical values, as in Equations (4).

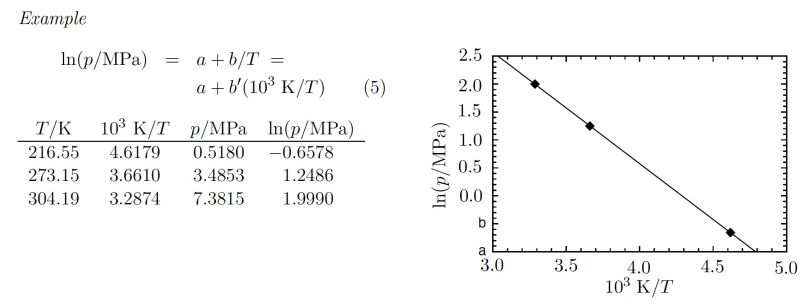
Algebraically equivalent forms may be used in place of $10^3\ \rm{K/T}$ , such as $\rm{kK}/T$ or $10^3\ (T/\rm{K})^{−1}$. Equations between numerical values depend on the choice of units, whereas equations between quantities have the advantage of being independent of this choice. The method described here for handling physical quantities and their units is known as quantity calculus (see ISO [5.a] and [11–14]). It is recommended for use throughout science and technology. The use of quantity calculus does not imply any particular choice of units (see Section 3.1, p. 15 for quantity calculus).

1.2 BASE QUANTITIES AND DERIVED QUANTITIES

By convention physical quantities are organized in a dimensional system built upon seven base quantities, each of which is regarded as having its own dimension. These base quantities in the International System of Quantities (ISQ) on which the International System of units (SI) is based, and the principal symbols used to denote them and their dimensions are as follows:

• • Insert Table**

All other quantities are called derived quantities and are regarded as having dimensions derived algebraically from the seven base quantities by multiplication and division.

Example: dimension of energy is equal to dimension of $M\ L^2\ T^{−2}$. This can be written with the symbol dim for dimension (see footnote 1, below) $\dim(E) = \dim(m·l^2·t^{−2}) = M\ L^2\ T^{−2}$

The quantity amount of substance is of special importance to chemists. Amount of substance is proportional to the number of specified elementary entities of the substance considered. The proportionality factor is the same for all substances; its reciprocal is the Avogadro constant (see Section 4.10, p. 44). The SI unit of amount of substance is the mole. "Amount of substance" is also called “chemical amount”, and may be abbreviated to the single word "amount", particularly in such phrases as “amount concentration” (see footnote 2), and "amount of $\ce{N2}$". In the same sense one might for instance say “amount concentration of $\ce{N2}$.” A possible name for international usage has been suggested: “enplethy” [10] (from Greek, similar to enthalpy and entropy).

In the ISQ, electric current is chosen as base quantity and ampere is the SI base unit. In atomic and molecular physics, the so-called atomic units are useful (see Section 2.4, p. 12).

Ordinal quantities and nominal properties are outside the scope of the Green Book [9].